
| Home |
| The WEB-Method |
| Examples |
| Publications |
| Contact |
Scattered Data Approximation
WEB-splines settle a notorious problem in scattered data approximation: If the data sites are given on a non-rectangular domain, then the standard B-spline approximation typically reveals shape artifacts at grid cells with few data points. This undesired behavior is avoided when web-splines are used as approximation space.
As an example, we consider scattered data sampled from a smooth function. The grid lines are non-uniform and adapted to the structure of the domain.
data sites and grid lines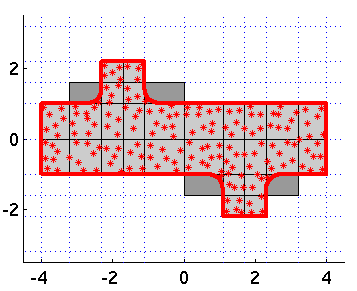 |
function to be approximated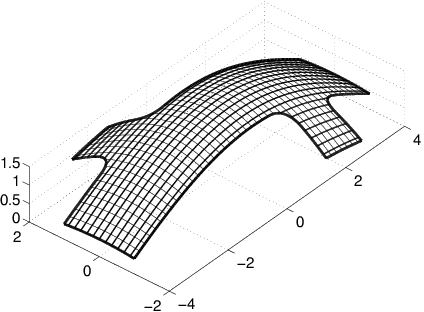 |
The standard B-spline approximation yields a large deviation near the boundary due to the instability of the basis. By contrast, the web-spline approximation is perfectly smooth and its maximal deviation from the given function is significantly smaller.
standard approximation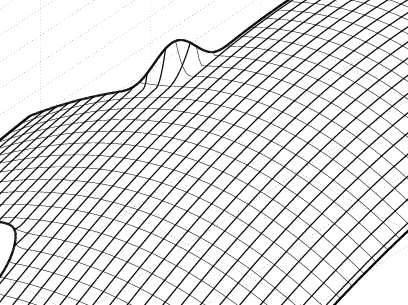 maximum error = 0.3 |
web-spline approximation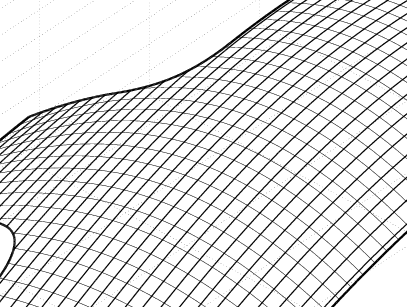 maximum error = 0.0002 |
Literature
K. Höllig, U. Reif: Nonuniform Web-Splines. Computer Aided Geometric Design, Volume 20, Issue 5, pp. 277-294, 2003.See Publications for a complete list of WEB-publications.
Author: Ulricht Reif ; Last modification: 2003/10/29 08:36:58 UTC.
| [Home] [The WEB-Method] [Examples] [Publications] [Contact] |